Probability is a measure of the likeliness that an event will occur. Odds are a way of expressing probability.
Typically probability refers to the likely-hood or chance that something will happen. However, we can also measure the odds or probability that something won’t occur. Odds and probability are always a theoretical measure of chance as opposed to a guarantee that something will happen. Probability and odds are calculated mathematically, but not all factors related to a final equation are rooted in mathematics (such as human behavior, legislation, and changes in weather patterns).
Probability and odds are vital to fields of statistics, mathematics, finance, gambling, science, artificial intelligence, computing, game theory, philosophy, healthcare, security, weather, big data, and more. When people think of odds, they may think of “the odds of winning the lotto”, but the statistics based skill-set behind probability and odds have far reaching applications.
How to Express Probability Statistics: In statistics the probability (or “chance”) of rolling a 6 on a six sided die can be expressed as 1 in 6, 1/6, or 16.7%.
How to Express Odds in Statistics: The odds-for can be expressed as 1 to 5 (we expect 1 six event for ever 5 non-six events), and likewise the odds-against can be expressed as 5 to 1.
How to Express Odds in Gambling: In gambling odds can mean “payout” or probability. So in gambling 5 to 1 either means it pays $5 for every $1 bet. Or it means a 5 in 1 chance of happening (just like we express probability in statistics).
In every day language odds and probability are both used interchangeably to refer to “probability” or “chance”.
Whatever is the case, is the case. That is to say, whatever is true is true within a system, is true within that system (for example, whatever is the case, in reality, is the case in reality; or, whatever is true in mathematics, is true in mathematics).
Water is continuously mixed and recycled, so the odds are in favor of us sharing at least some molecules of it with Socrates, Jesus, Buddha, or anyone from past eras.
According to 2016 data, slot machines account for more revenue than all other casino games combined, and penny slots account for more revenue than any other casino game.
The law of large numbers says, the more instances of a probable event that are considered, the more the theoretical and actual results converge.
Blaise Pascal and Pierre de Fermat invented probability theory in 1654 to solve a gambling problem related to expected outcomes.
Human behavior can be random to some extent, but most behavior is based on prior input, and thus is “deterministic” (meaning not totally random).
Everything is either true or not true, but not everything that is true can be proven true, and not everything false can be proven false.
Very few can make a living off of blackjack. To win in the long run you must count cards, watch tables, risk big money, and employ questionable betting strategies.
In March of 2016, Google’s AI AlphaGo beat Lee Sedol, one of the best professional Go players in the world, in four out of five games in a no-mercy match.
Light is a particle (a photon), that acts like a wave (“both a particle and a wave”), which can be measured as an excited quantized state of the electromagnetic field.
General systems theory shows us there are commonalities between different types of physical and conceptual systems which we can study to better understand complex systems.
Bayes’ theorem is a probability theory used to calculate the likelihood of an event being true or not true based on conditions related to the event. (i.e. an equation used for calculating conditional probabilities).
Correlation does not imply causation, but it can indicate it. The more correlating factors between events, the more likely there is a causal relationship.
Past results of random independent events, like a coin flip, don’t affect future results. The mistaken belief that past results affect future results is known as “the Gambler’s Fallacy” (AKA the Fallacy of the Maturity of Chances, or the Monte Carlo Fallacy).
Quantum computers, unlike classic computers, aren’t limited to binary bits (which are always 0 or 1), they use qubits (which can be 0 and 1 at the same time).
If you build it, you increase the odds of them coming. In other words, if you put thought and energy into a project you increase the likelihood of success.
Computers can’t generate truly random numbers in the purest sense with software alone. However, computers can generate truly random numbers with the help of natural random events.
Most people have about 6 degrees of separation (small world theory), but not everyone has 6 degrees of separation from Kevin Bacon or any other given person.
We explain inductive reasoning, a bottom-up reasoning method that reasons by consistency, comparing particulars and probabilities to find likely truths.
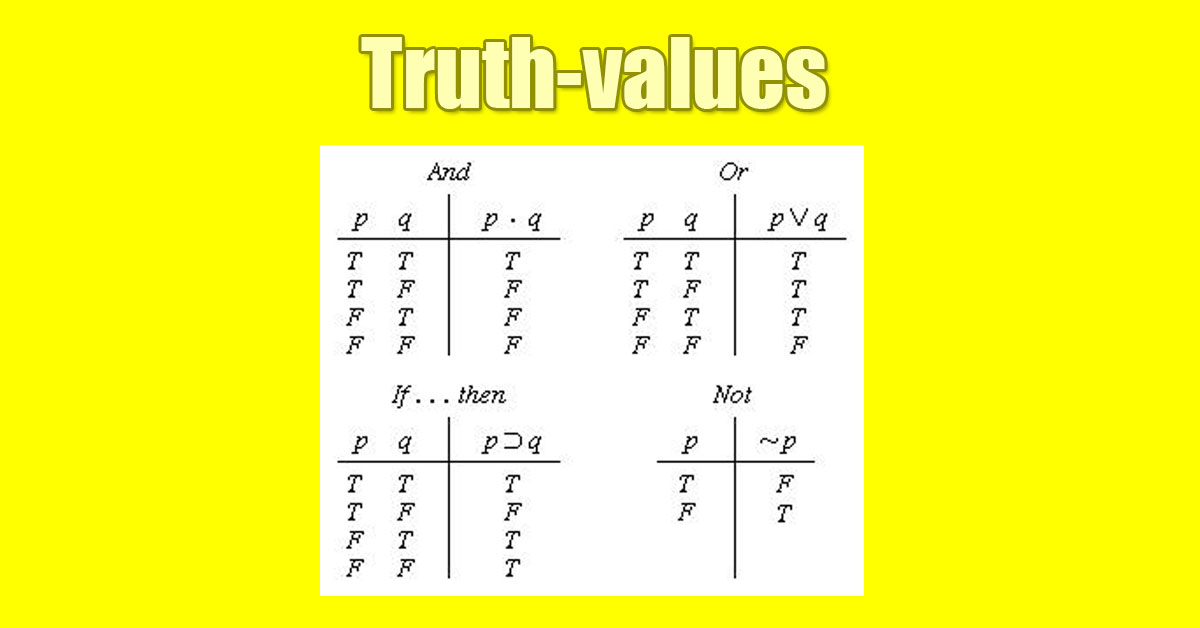
A truth-value is a label that is given to a statement (a proposition) that denotes the relation of the statement to truth.
In practice, human action often has paradoxical or unintended effects. Sometimes effects or side effects even have the exact opposite effect as intended.
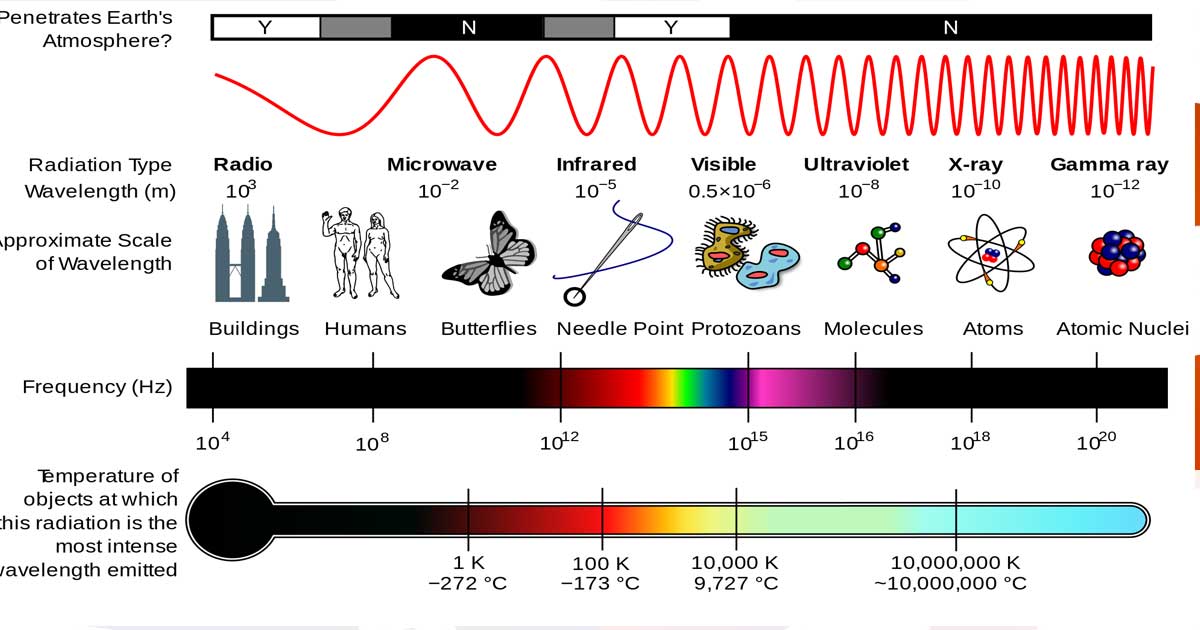
We explain “light,” both as electromagnetic radiation within a visible portion of the electromagnetic spectrum, and as electromagnetic energy carried by photons.
By continuing to use the site, you agree to the use of cookies. more information
The cookie settings on this website are set to "allow cookies" to give you the best browsing experience possible. If you continue to use this website without changing your cookie settings or you click "Accept" below then you are consenting to this.