Reference Frames Examples (Physics)

Reference Frames and Relativity Explained in Simple Terms, Using Examples, and Without Math

Reference frames and relativity in physics explained using math-free examples. We cover frames of reference, inertial frames, accelerated frames, and relativity.

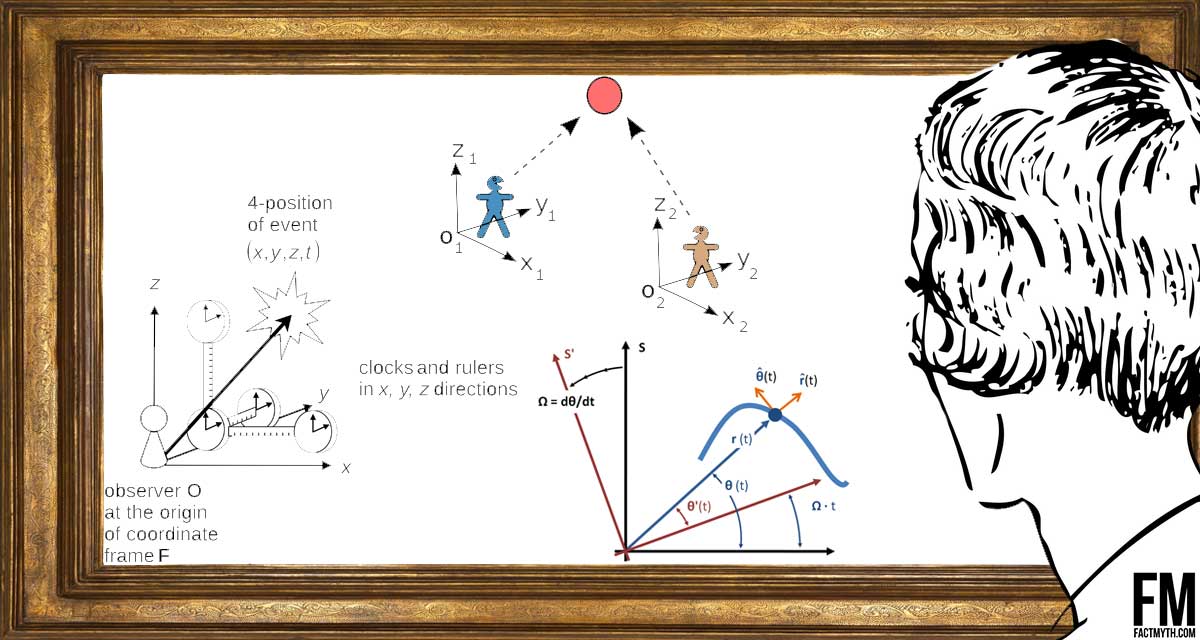
What is a reference frame? A reference frame is simply a perspective, context, or point of view. In physics that typically means physical space time coordinates, in the social sciences it takes on a slightly different meaning. In both fields, everything is relative to the point of view of the observer (relativity). This pages focuses on reference frames in physics, see a general overview of reference frames.

TIP: The video below is probably the coolest reference frame video of all time. I would watch this first.

Frames of Reference (1960) Educational Film. In simple terms, you can’t accurately judge something until you have something to compare it to.

Defining Some Physics Terms

We will assume you know some reference frame basics for the examples below, but here is a quick overview of terms you need to know.

• Frame of reference: A point of view, context, or set of coordinates with which we can orient ourselves. This can be a person’s physical viewpoint or can be space-time coordinates we calibrate an instrument to. It is a defined vantage-point that we can observe/measure from.
• Inertial Frame: Any frame of “relative rest” like we have standing still on earth. Any frame of relative rest, regardless of what speed the frames are moving, is an inertial frame. It is where “the laws of inertia” or Newton’s Laws of motion like F=ma: force = mass times acceleration apply.
• Accelerated Frame: A frame which is accelerating away from what is being observed. A frame not at “relative rest”, so a non-inertial frame. We need to account for special relativity in frames of non-rest like this.
• The observer: That which is “looking through the frame.” If we think of the frame as a lens, then the observer is the lens or the act of looking through the lens.
• Relativity: The fact that all motion, time, space, and such is relative to the observer’s frame of reference.
• Constants: The things in the universe that aren’t relative. See a list of constants.

Special Relativity | Lecture 1. Leonard Susskind is teaching a course at Stanford. If you have two hours, I suggest watching this video. Reference frames can be understood in the context of special relativity.

TIP: We take a much different approach, but if you want another angle, see Study Physic’s Lesson 9: Relative Motion and Frames of Reference.

Reference Frames and Relativity Example 1

The content below explains why reference frames are important, and how they apply to the physical universe. There are lots of different ways to approach the subject, but generally these are at least intermediate concepts and take time to learn. We also explain the different types of relativity as they are closely related to reference frames. See the aforementioned video above for a simple and professional explainer.

TIP: The below example ignores nitpicking things like our ability to sense motion, our ability to look at ourselves as a reference point, and the fact that we can’t see without a light source (which on some level would also be a reference point).

0 Reference Points

Imagine the blackness of space with no gravity, landmark, or frame of reference. There is nothing observing this true empty space. There is no observer and no reference frame, and thus, nothing can be known. Without something, there is very literally nothing (get semi-heady physics explainers of this here).

1 Reference Point

Now let’s put ourselves in the empty space. Now there is us, and nothing else. Our single reference frame (our perception) is totally useless as nothing is happening aside from us observing empty space. We can’t tell if we are moving, or in what direction, as there are no additional reference point to determine spin, momentum, acceleration, etc (here we assume there is no force acting on us, thus we can’t use our internal senses for orientation).

Inertial Frames and Relative Motion

Now we see a space friend floating next to us, moving the same speed and in the same direction as we are. Our friend gives us another reference point. However, he or she is floating next to us at relative rest in an “inertial frame.” Given this, we can’t tell if we are inactive or moving due to Galilean Relativity, i.e. relative motion. In this situation, our company is useless, but at least we have a friend, so we can start speculating more than just “I think therefore I am” (René Descartes).

TIP: Next we get into relativity. It isn’t hard, but it is full of potentially new concepts. I suggest the video below to supplement your reading.

Are Space and Time An Illusion? | Space Time | PBS Digital Studios. If you need some other “frame of reference” for understanding relativity I strongly suggest one of the best series on the internet PBS Spacetime. Specifically see PBS Spacetime’s relativity playlist.

Accelerated Frames, Special Relativity, and General Relativity

Now, suddenly our friend and I both start accelerating away from each other (accelerated frame). Despite this new variable, neither can tell who is moving and who is staying still without additional landmarks (AKA coordinates, AKA reference points). If we look only at each other, we can only determine relative motion (between our two bodies), we still can’t tell which body is experiencing motion (still Galilean Relativity, i.e. relative motion).

As we accelerate away from each other time dilates and length contracts (Special Relativity). Not only can we not tell who is moving, we see time slow down for the other person and see them shrink yet we experience time and length normally ourselves. If we asked our friend what was happening, they would give us a very different account because special relativity is strange like that giving each observer a different perspective from their frame of reference. So instead of clarifying what happened, we just got more confused.

Now, let us use our imagination to make things even more confusing. There is now a giant invisible mass (dark matter) near us. It isn’t giving us a reference point as we don’t have tools to measure it, but it is dilating time and space, and curving spacetime just like accelerating did (General Relativity).

At this point, we can’t tell who is moving. Instead of clarifying what is happening, we have shown that time and length are relative to the observer’s reference frame and speed, as well as proximity to mass. Thus, not only can we not tell what is actually happening, we don’t even agree with our space-friend on what seems to be happening.

To add one last point of confusion, we can’t even confirm we are moving because there is an infinite distance in-between any two points in spacetime. Luckily, the laws of physics are here to help.

The Laws of Physics and the Constants

First off, Albert Einstein tells us that “the laws of physics are the same in all inertial frames of reference” or stationary reference frames like those we have on earth.

Secondly, also thanks to Einstein and other scientists such as Maxwell, we can tell a few things from the universal constants. We know that “the speed of light in a vacuum” is constant. Spacetime (three dimensions of space and one dimension of time as a single construct) is constant. The gravitational force between two bodies is constant (that one is Newton’s). We also know that time and space quantize to Planck’s constant, so we can accelerate through them without worrying about infinity.

Having these constants, we can do some math and show that both our friend and we are moving at a speed relative to light. We move in relative Plank lengths of time forward. When we accelerate, the extra energy from acceleration increases our relativistic-mass causing spacetime to curve around us the same way that proximity to mass does. This “speeds up” our relative time, which other people, from a less massive or less accelerated frame, would see as time moving slowly. We realize this effect is happening to both our space friend and us. Now we can figure out what is going on. With enough physical and conceptual reference points, we were able to piece together the puzzle.

You don’t have to understand all the above, just know, that each reference point we use gives us another angle for understanding the true cause and effect relationships in the universe.  Limits and constants can be used as “coordinates” in our reference frames for measurement. So even though everything we observe depends on the frame of reference, we can still work out the truth using our knowledge!

Introduction to Reference Frames (Physics).

FACT: In the physical universe all systems are made of elementary particles and all systems follow the same core set of rules. To determine any of this from within, or without the system, requires reference frames.

TIP: If you have a “bad” reference frame, life gets really confusing quickly. When we look up at other planet’s orbits from Earth, it seems as though they are moving in random patterns. Our frame of reference isn’t useful for tracking orbits. If we zoom up and take a nice big section of spacetime as our reference frame, we see that gravity is simply making all the planets orbit around the sun. We can’t see that from our perspective because we are spinning around in circles. If we factor out our spin, the picture becomes clearer.

Reference Frames and Relativity Example

If I’m sitting under a tree and an apple falls on my head, from my perspective, the apple fell on my head (because things fall downward on earth). If we ask Galileo he says, “we can’t tell if it’s us or the apple that is falling, all motion is relative.”

If we ask Newton he says, “objects in motion tend to stay in motion,” “the force the apple hits us with is equatable to the mass of the apple times it’s acceleration” (F=ma), and “each force has an equal and opposite force.” This helps us paint a clearer picture. Newton called this concept “Gravity.” In Newton’s terms “all objects with mass simply fall toward each other with acceleration proportional to their mass.”

Given this, we can calculate force, mass, and acceleration of objects but we don’t have the full picture. Newton assumed that the acceleration from gravity was a constant force acting on all bodies with mass. If we zoom  out into space with our magic perspective camera and look at our spinning orbiting earth, it looks as though the earth is accelerating pushing our head toward the apple, and the earth toward our feet at 9.80665 m/s².

But if we ask Einstein he says, “gravity is just the curvature of spacetime by things with mass-energy pushing us against the ground with force relative to the mass and acceleration of the earth.” Einstein also said, “the laws of physics are the same in all inertial frames of reference” (frames of a relative rest), “the speed of light is constant,” and “spacetime is constant.”

If we put this all together, it means that there is no difference between what is happening on earth with the apple, and what would happen in a magic space elevator accelerating at 9.80665 m/s². In other words, the inertial F=ma mass is analogous in any inertial reference frame.

The grand result: Spacetime is curving around us and holding us on the earth, but how we perceive this depends on our frame of reference. An apple “falling” on our head on earth, or an apple accelerating toward our head at 9.80665 m/s² in space, or us accelerating toward the apple at the same speed, or us and the apple moving at each other at half that speed, etc. are all analogous. All of this can be measured with F=ma and a little math because it is all the same effect, which is spacetime curvature from mass-energy. Really, it depends on frame of reference.

Frames of Relative Motion

Now, still looking at the above example, if we move at a frame of relative motion (not rest), things get tricky. At higher speeds, mass will increase due to the kinetic energy needed to accelerate the mass (F=ma). We can calculate the extra energy using Einstein’s mass energy equivalence equation, so while this can be accounted for in the measurement process, the effects are very real.

If we look through our imaginary perspective camera, which is accelerating away from the apple hitting our head, we see two things. Firstly, the apple is falling more slowly. Secondly, both we and the apple are getting smaller.

With relative frames of motion, time dilates, length contracts, and stuff gets strange. This is because everything happening in the universe is relative to everything else aside from constants like the speed of light in a vacuum, spacetime, and the “Planck length.”

Since we know everything is relative, we must give ourselves limits just like limits in calculus.

Universal Limits (Coordinates)

Luckily, as noted above, the universe has natural limits (physical constants) like light speed, the Planck length, and importantly three dimensions of space and one dimension of time. It also has natural rules like F=ma, E=mc², and the laws of conservation. Knowing all this we can draw “coordinates” to ensure that we can understand things from our perspective. We can measure things from a universal perspective based on cause and effect relationships happening at light speed.

When we truly zoom out, we see spacetime is curving due to mass-energy, and everything is ACTUALLY happening at different speeds relative to the speed of light. We can use an E=mc² to account for acceleration, and measure everything from Newton’s original F=ma from all inertial frames. When we combine that with Einstein’s relativity, we get a much clearer understanding of how the universe works, and why it looks so odd from some perspectives.

Intro to Einstein’s Special Relativity | Doc Physics.